Calculus is a limb of maths centred on points of confinement, methods, derivatives, integrals, and unbounded sequence. This subject constitutes a major part of up to date arithmetic training. It has two major limbs, differential maths and necessary analytic, which are identified by the basic theorem of analytic. Maths is the investigation of change, in the same way that geometry is the investigation of shape and variable based maths is the investigation of operations and their requisition to understanding mathematical statements. A course in analytic is a portal to different, more progressed courses in maths dedicated to the investigation of capacities and points of confinement, broadly called scientific investigation.
Calculus has boundless requisitions in science, mass trading, and building and can tackle a large number of situations for which polynomial math apart from everyone else is lacking.
Related posts:
Because of shifts in naming gatherings, and the whims of the cartridge makers, shot widths can differ substantially from the width suggested by the name. Case in point, there is a departure of the same amount as 0.045 creeps (1.15 mm) between the most diminutive and most impressive of the some cartridges designated as '.38 bore'. Then again it might be noted that .38 crawls is more than 9 1/2 mm. ...
The experimental investigation of probability is a current infrastructure. Betting demonstrates that there has been an investment in quantifying the thoughts of chance for centuries, anyway correct scientific depictions emerged much later. There are explanations obviously, for the moderate improvement of the arithmetic of chance. While diversions of chance furnished the impulse for the numerical i...
Unique algebra based maths was upgraded in the 19th century, deriving from the premium in handling examinations, from the get go fixating on what is now called Galois speculation, and on constructibility issues. The "present day polynomial maths" has significant nineteenth-century creates in the work, for example, of Richard Dedekind and Leopold Kronecker and critical interconnections with diverse...
Differential calculus is the study of the definition, lands, and requisitions of the derivative of a method. The procedure of discovering the derivative is called differentiation. Given a role and a focus in the realm, the derivative at that indicate is a way of encoding the modest-scale conduct of the role close to that indicate. By discovering the derivative of a capacity at each focus in its sp...
Arithmetical geometry is a limb of math, traditionally considering lands of the sets of zeros of polynomial mathematical statements. Advanced logarithmic geometry is dependent upon additional conceptual procedures of unique polynomial math, in particular commutative polynomial math, with the dialect and the situations of geometry.
The saying statistics, when pointing to the experimental train, is solitary in "Statistics is an art." This might as well not be confounded with the expression statistic, pointing to an amount (for example mean or average) figured from a set of data, whose plural is statistics ("this statistic appears wrong" or "these statistics are misdirecting").
In calculus, foundations points to the thorough advancement of a subject from exact adages and definitions. In promptly calculus the utilization of microscopic amounts was thought unrigorous, and was furiously condemned by various creators, most outstandingly Michel Rolle and Priest Berkeley. Berkeley popularly depicted infinitesimals as the phantoms of withdrew amounts in his book The Investigato...
Some acknowledge statistics to be a scientific collection of science relating to the accumulation, examination, elucidation or clarification, and presentation of data, while others recognize it a limb of mathematics concerned with gathering and deciphering information. Due to its experimental roots and its center on requisitions, statistics is typically acknowledged to be a different numerical sci...
In current maths, the foundations of calculus are incorporated in the field of veritable dissection, which holds full definitions and confirmations of the theorems of calculus. The achieve of calculus has moreover been significantly amplified. Henri Lebesgue developed measure speculation and utilized it to outline integrals of all but the most obsessive roles. Laurent Schwartz presented Conveyance...
The formal investigation of calculus consolidated Cavalieri's infinitesimals with the math of limited divergences advanced in Europe at around the same time. Pierre de Fermat, guaranteeing that he acquired from Diophantus, presented the idea of adequality, which acted for fairness up to a minute failure term. The synthesis was attained by John Wallis, Isaac Pushcart, and James Gregory, the last tw...
Algebra based math is identified with arithmetic, be that as it may for recorded explanations, the saying "polynomial math" has several significances as a uncovered word, hinging on the connection. The saying in addition constitutes different terms in science, demonstrating more change in the significance. This article gives a wide outline of them, incorporating the history.
Integral calculus is the investigation of the definitions, lands, and provisions of two identified ideas, the uncertain essential and the unambiguous vital. The procedure of discovering the quality of an indispensable is called incorporation. In specialized dialect, basic analytics studies two identified direct specialists.
The Global Framework of Units (abridged SI from French: Système universal d'unités) is the advanced type of the metric framework. It involves a framework of units of estimation devised around seven base units and the benefit of the number ten. The SI was built in 1960, in view of the metre-kilogram-second framework, instead of the centimetre-gram-second framework, which, in turn, had a few variant...
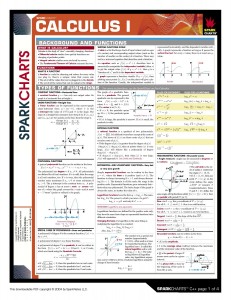
The image shows the most used abbrevations and most used equations in the Mathematics.
In arithmetic, a twofold connection on a set An is an accumulation of requested matches of components of A. In different expressions, its a subset of the Cartesian feature A2 = A × A. Ordinarily, a binary connection between two sets An and B is a subset of A × B. The terms dyadic connection and 2-place connection are synonyms for double relations. An illustration is the "partitions" connection...
Calculus is a limb of science centered on breaking points, methods, derivatives, integrals, and endless arrangement. This subject constitutes a major part of current science instruction. It has two major limbs, differential maths and vital analytics, which are identified by the central theorem of maths. Math is the investigation of modification, in the same way that geometry is the investigation o...
The adjective "algebraic" regularly denotes connection to digest polynomial math, as in "mathematical structure". In any case in certain cases it points to mathematical statement explaining, reflecting the advancement of the field. Rudimentary polynomial math, regularly part of the curriculum in optional instruction, presents the notion of variables speaking for numbers. Proclamations dependen...
Calculus has generally been called "the math of infinitesimals", or "minute analytics". For the most part, analytics (plural calculi) points to any system or framework of count guided by the symbolic control of declarations. Certain samples of different well-known calculi are propositional analytics, variational math, lambda math, pi analytics, and unite math.

 Upload your infographic here and contribute to our community.
Upload your infographic here and contribute to our community. 
Leave a Reply