Upload Your Infographic
 InfoHow.org
InfoHow.org
Categories
- Advice
- Animals
- Architecture
- Arts
- Banking
- Bitcoin & Blockchain
- Business, Finance & Employment
- Companies
- Countries, Maps & Geography
- Education
- Electronics & Technology
- Entertainment
- Fashion, Clothing & Grooming
- Food & Drink
- Holidays
- How To
- Internet & Communication
- Law & Crime
- Popular Culture & Social Media
- Science
- Social Science
- Society, Culture & Relationships
- Sports & Health
- Statistics
- Survival DIY
- War, Weapons & Military
Math Archive
How to do Partial Fraction Decomposition?
Partial Fraction Decomposition is an algebraic technique to convert a complex rational function into sum of simple rational fractions. A rational function is the division of two polynomials. In some […]
QS Statistics (4)
Some acknowledge statistics to be a scientific collection of science relating to the accumulation, examination, elucidation or clarification, and presentation of data, while others recognize it a limb of mathematics […]
SC Calculus I (4)
In calculus, foundations points to the thorough advancement of a subject from exact adages and definitions. In promptly calculus the utilization of microscopic amounts was thought unrigorous, and was furiously […]
Card Counting
Card Counting is a club card event methodology utilized fundamentally within the blackjack group of clubhouse recreations to certify if the subsequently hand is possible to give a feasible playing […]
SC Algebra I (2)
The adjective “algebraic” regularly denotes connection to digest polynomial math, as in “mathematical structure”. In any case in certain cases it points to mathematical statement explaining, reflecting the advancement of […]
International System of Units Prefixes
The Universal Framework of Units (condensed SI from French: Système worldwide d’unités) is the advanced manifestation of the metric framework. It contains a framework of units of estimation devised around […]
Metric Conversion Chart
The Global Framework of Units (abridged SI from French: Système universal d’unités) is the advanced type of the metric framework. It involves a framework of units of estimation devised around […]
RS Calculus Integrals
Calculus Integrals is a significant notion in arithmetic and, as one with its converse, differentiation, is one of the two primary operations in analytics. Given a capacity f of a […]
Russian Multiplication
In arithmetic, antiquated Egyptian duplication (likewise reputed to be Egyptian augmentation, Ethiopian duplication, Russian increase, or worker increase), one of two augmentation techniques utilized by recorders, was a methodical system […]
SC Calculus II (2)
In current maths, the foundations of calculus are incorporated in the field of veritable dissection, which holds full definitions and confirmations of the theorems of calculus. The achieve of calculus […]
SC Calculus Reference (2)
Integral calculus is the investigation of the definitions, lands, and provisions of two identified ideas, the uncertain essential and the unambiguous vital. The procedure of discovering the quality of an […]
SC Calculus Reference (1)
Differential calculus is the study of the definition, lands, and requisitions of the derivative of a method. The procedure of discovering the derivative is called differentiation. Given a role and […]
SC Calculus II (5)
In the 19th century, infinitesimals were traded by breaking points. Breaking points depict the quality of a method at a certain include in terms of its qualities at nearby enter. […]
SC Calculus II (4)
Calculus is more often than not advanced by controlling exceptionally modest amounts. Truly, the first technique for doing so was by infinitesimals. These are questions which might be treated like […]
SC Calculus II (3)
Limits points are not the sole meticulous way to the organization of calculus. An elective is Abraham Robinson’s non-standard dissection. Robinson’s methodology, improved in the 1960s, utilizes specialized apparatus from […]
SC Calculus II (1)
Numerous mathematicians, incorporating Maclaurin, tried to confirm the soundness of utilizing infinitesimals, yet it could not be until 150 years later when, because of the work of Cauchy and Weierstrass, […]
SC Calculus I (3)
The formal investigation of calculus consolidated Cavalieri’s infinitesimals with the math of limited divergences advanced in Europe at around the same time. Pierre de Fermat, guaranteeing that he acquired from […]
SC Calculus I (2)
Calculus has generally been called “the math of infinitesimals”, or “minute analytics”. For the most part, analytics (plural calculi) points to any system or framework of count guided by the […]
SC Calculus I (1)
Calculus is a limb of maths centred on points of confinement, methods, derivatives, integrals, and unbounded sequence. This subject constitutes a major part of up to date arithmetic training. It […]
SC Algebra I (4)
Unique algebra based maths was upgraded in the 19th century, deriving from the premium in handling examinations, from the get go fixating on what is now called Galois speculation, and […]
SC Algebra I (3)
The saying algebra based math hails from the Arabic dialect and much of its techniques from Arabic/Islamic science.
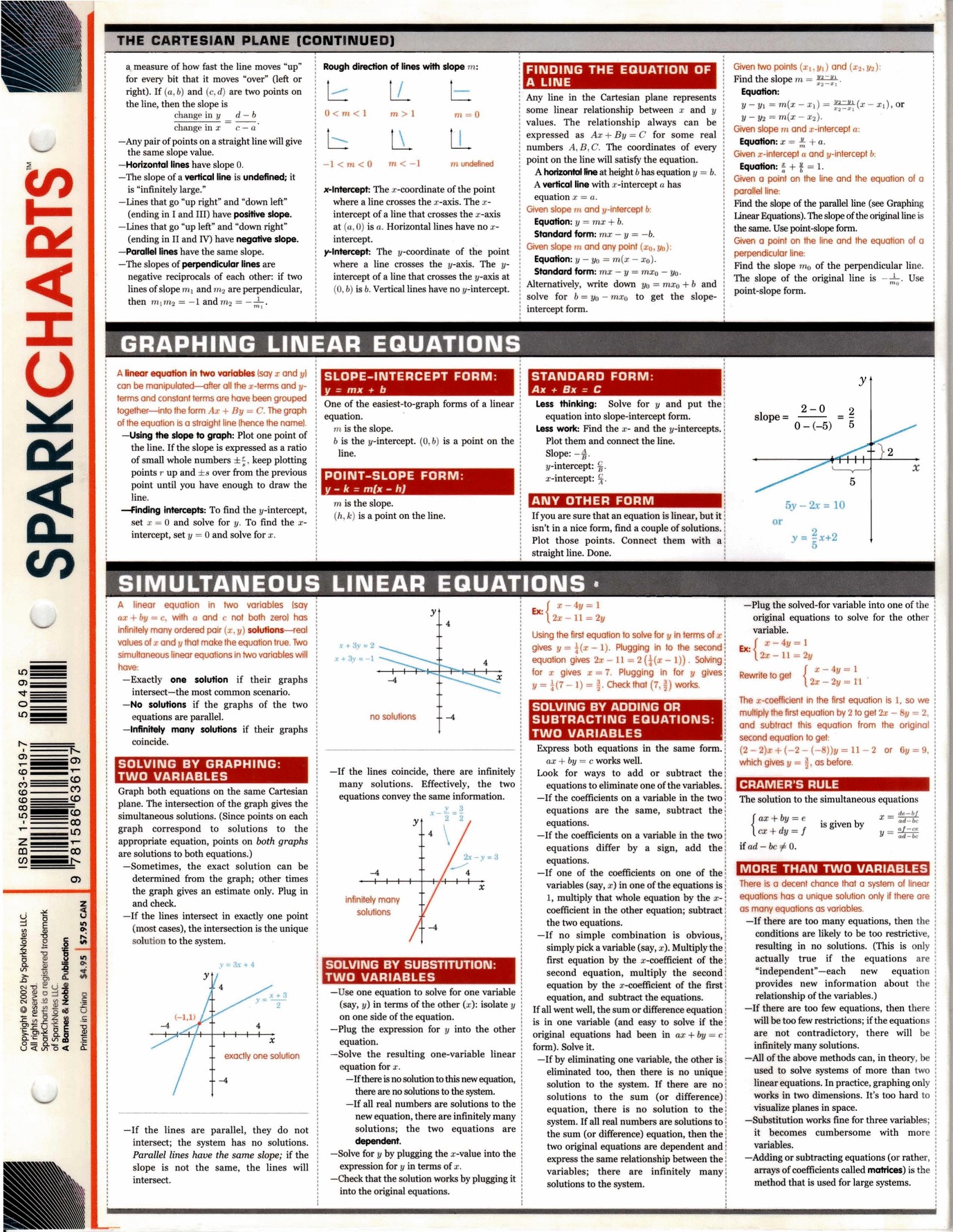
SC Algebra I (1)
Algebra based math is identified with arithmetic, be that as it may for recorded explanations, the saying “polynomial math” has several significances as a uncovered word, hinging on the connection. […]
RS Trigonometry – Definition
Trigonometry nuts and bolts are regularly showed in school either as a unattached course or as a component of a precalculus course. The trigonometric roles are pervasive in parts of […]
RS Geometry – Shapes & Solids
Geometry is an extension of science concerned with issues of shape, size, relative position of figures, and the lands of space. A mathematician who works in the field of geometry […]

![How to do Partial Fraction Decomposition? Partial Fraction Decomposition is an algebraic technique to convert a complex rational function into sum of simple rational fractions. A rational function is the division of two polynomials. In some […]](https://www.infohow.org/wp-content/uploads/2016/11/repeating-factor-partial-fraction-updated1-1-195x110.png)
![QS Statistics (4) Some acknowledge statistics to be a scientific collection of science relating to the accumulation, examination, elucidation or clarification, and presentation of data, while others recognize it a limb of mathematics […]](https://www.infohow.org/wp-content/uploads/2012/11/QS-Statistics-4.jpg)
![SC Calculus I (4) In calculus, foundations points to the thorough advancement of a subject from exact adages and definitions. In promptly calculus the utilization of microscopic amounts was thought unrigorous, and was furiously […]](https://www.infohow.org/wp-content/uploads/2012/11/SC-Calculus-I-4.jpg)
![Card Counting Card Counting is a club card event methodology utilized fundamentally within the blackjack group of clubhouse recreations to certify if the subsequently hand is possible to give a feasible playing […]](https://www.infohow.org/wp-content/uploads/2012/11/Card-Counting.png)
![SC Algebra I (2) The adjective “algebraic” regularly denotes connection to digest polynomial math, as in “mathematical structure”. In any case in certain cases it points to mathematical statement explaining, reflecting the advancement of […]](https://www.infohow.org/wp-content/uploads/2012/11/SC-Algebra-I-2.jpg)
![International System of Units Prefixes The Universal Framework of Units (condensed SI from French: Système worldwide d’unités) is the advanced manifestation of the metric framework. It contains a framework of units of estimation devised around […]](https://www.infohow.org/wp-content/uploads/2012/11/International-System-of-Units-Prefixes.png)
![Metric Conversion Chart The Global Framework of Units (abridged SI from French: Système universal d’unités) is the advanced type of the metric framework. It involves a framework of units of estimation devised around […]](https://www.infohow.org/wp-content/uploads/2012/11/Metric-Conversion-Chart.png)
![RS Calculus Integrals Calculus Integrals is a significant notion in arithmetic and, as one with its converse, differentiation, is one of the two primary operations in analytics. Given a capacity f of a […]](https://www.infohow.org/wp-content/uploads/2012/11/RS-CalculusIntegrals.gif)
![Russian Multiplication In arithmetic, antiquated Egyptian duplication (likewise reputed to be Egyptian augmentation, Ethiopian duplication, Russian increase, or worker increase), one of two augmentation techniques utilized by recorders, was a methodical system […]](https://www.infohow.org/wp-content/uploads/2012/11/Russian-Multiplication.gif)
![SC Calculus II (2) In current maths, the foundations of calculus are incorporated in the field of veritable dissection, which holds full definitions and confirmations of the theorems of calculus. The achieve of calculus […]](https://www.infohow.org/wp-content/uploads/2012/11/SC-Calculus-II-2.jpg)
![SC Calculus Reference (2) Integral calculus is the investigation of the definitions, lands, and provisions of two identified ideas, the uncertain essential and the unambiguous vital. The procedure of discovering the quality of an […]](https://www.infohow.org/wp-content/uploads/2012/11/SC-Calculus-Reference-21.jpg)
![SC Calculus Reference (1) Differential calculus is the study of the definition, lands, and requisitions of the derivative of a method. The procedure of discovering the derivative is called differentiation. Given a role and […]](https://www.infohow.org/wp-content/uploads/2012/11/SC-Calculus-Reference-11.jpg)
![SC Calculus II (5) In the 19th century, infinitesimals were traded by breaking points. Breaking points depict the quality of a method at a certain include in terms of its qualities at nearby enter. […]](https://www.infohow.org/wp-content/uploads/2012/11/SC-Calculus-II-5.jpg)
![SC Calculus II (4) Calculus is more often than not advanced by controlling exceptionally modest amounts. Truly, the first technique for doing so was by infinitesimals. These are questions which might be treated like […]](https://www.infohow.org/wp-content/uploads/2012/11/SC-Calculus-II-41.jpg)
![SC Calculus II (3) Limits points are not the sole meticulous way to the organization of calculus. An elective is Abraham Robinson’s non-standard dissection. Robinson’s methodology, improved in the 1960s, utilizes specialized apparatus from […]](https://www.infohow.org/wp-content/uploads/2012/11/SC-Calculus-II-3.jpg)
![SC Calculus II (1) Numerous mathematicians, incorporating Maclaurin, tried to confirm the soundness of utilizing infinitesimals, yet it could not be until 150 years later when, because of the work of Cauchy and Weierstrass, […]](https://www.infohow.org/wp-content/uploads/2012/11/SC-Calculus-II-1.jpg)
![SC Calculus I (3) The formal investigation of calculus consolidated Cavalieri’s infinitesimals with the math of limited divergences advanced in Europe at around the same time. Pierre de Fermat, guaranteeing that he acquired from […]](https://www.infohow.org/wp-content/uploads/2012/11/SC-Calculus-I-3.jpg)
![SC Calculus I (2) Calculus has generally been called “the math of infinitesimals”, or “minute analytics”. For the most part, analytics (plural calculi) points to any system or framework of count guided by the […]](https://www.infohow.org/wp-content/uploads/2012/11/SC-Calculus-I-2.jpg)
![SC Calculus I (1) Calculus is a limb of maths centred on points of confinement, methods, derivatives, integrals, and unbounded sequence. This subject constitutes a major part of up to date arithmetic training. It […]](https://www.infohow.org/wp-content/uploads/2012/11/SC-Calculus-I-1.jpg)
![SC Algebra I (4) Unique algebra based maths was upgraded in the 19th century, deriving from the premium in handling examinations, from the get go fixating on what is now called Galois speculation, and […]](https://www.infohow.org/wp-content/uploads/2012/11/SC-Algebra-I-4.jpg)
![SC Algebra I (1) Algebra based math is identified with arithmetic, be that as it may for recorded explanations, the saying “polynomial math” has several significances as a uncovered word, hinging on the connection. […]](https://www.infohow.org/wp-content/uploads/2012/11/SC-Algebra-I-1.jpg)
![RS Trigonometry – Definition Trigonometry nuts and bolts are regularly showed in school either as a unattached course or as a component of a precalculus course. The trigonometric roles are pervasive in parts of […]](https://www.infohow.org/wp-content/uploads/2012/11/RS-Trigonometry-Definition.gif)
![RS Geometry – Shapes & Solids Geometry is an extension of science concerned with issues of shape, size, relative position of figures, and the lands of space. A mathematician who works in the field of geometry […]](https://www.infohow.org/wp-content/uploads/2012/11/RS-Geometry-Shapes-Solids.gif)
 Upload your infographic here and contribute to our community.
Upload your infographic here and contribute to our community.